

– The prisms are polyhedrons or objects with multiple flat faces. When the two bases of a prism are perfectly aligned and its faces are rectangles (perpendicular to the bases) it is a right prism, else it is an oblique. = 2 × ( 1 2 x b x h) + 2 × (l x s) + (l x b)Įxample: Calculate the surface area and volume of the following prism. Surface Area = Area of base triangles + Area of side parallelograms For example, when you cover a box in wrapping paper, then you should know its surface area to get an idea of the actual quantity of paper.
#Area of a prism how to#
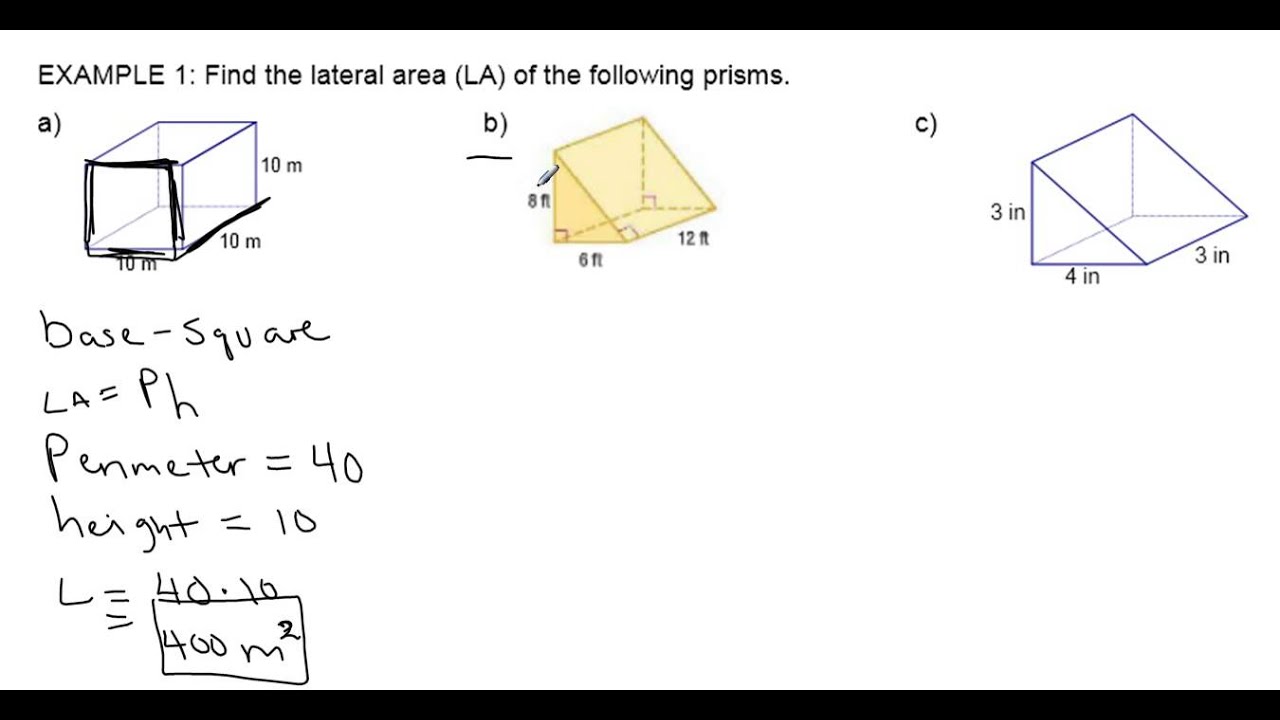
Let us see how to find the surface area and volume of a triangular prism. Surface area is the total space available outside of an object. Volume of a prism is the amount of space inside the prism. Find the area of the whole surface of a right triangular prism whose height is 36 m and the sides of whose bases are 51, 37 and 20 m, respectively. Rule 2: The total surface area of a prism is the sum of the lateral areas and the area of its base. The surface area of a prism is the sum of the area of all its faces. Rule 1: The lateral area of the prism is equal to the perimeter of the base times the altitude. Based on the shape of the base, prisms are regular or irregular prisms. The base of a prism can be a regular or irregular polygon. Lets build our equation for surface area of a rectangular prism starting with our formula: A 2 (wl + lh + hw) A 2 w l + l h + h w. The cross section of a prism parallel to the base of the prism is same as its base.

It is also referred to as the intersection of a plane with the three-dimensional object. The cross section of a geometric shape or an object is the shape obtained by cutting it straight. Other faces of a prism are parallelograms or rectangles.The bases can be a triangle, square, rectangle or any other polygon.These identical shapes are called “ bases”. A prism is a 3-dimensional shape with two identical shapes facing each other.Let us look at the surface area of the prism formula The lateral area is the area of the vertical faces, in case a prism has its bases facing up and down. So the surface area of this figure is 544. The total surface area of a prism is the sum of lateral surface area and area of two flat bases.
#Area of a prism plus#
So one plus nine is ten, plus eight is 18, plus six is 24, and then you have two plus two plus one is five. To open it up into this net because we can make sure We get the surface area for the entire figure. And then you have thisīase that comes in at 168. You can say, side panels, 140 plus 140, that's 280. Volume of a regular hexagonal prism 1-10 /11: Disp-Num 1 7 02:30 Under 20. 12 times 12 is 144 plus another 24, so it's 168. Calculates the volume and surface area of a regular hexagonal prism given the edge length and height. Region right over here, which is this area, which is Just have to figure out the area of I guess you can say the base of the figure, so this whole

It will have four rectangles that connect the. A trapezoidal prism is a three dimensional solid that has two congruent trapezoids for its top and lower base. And so the area of each of these 14 times 10, they are 140 square units. Use this area of trapezoidal prism calculator to find the area by using length of the top, length of the bottom and height values of trapezoidal prism. Now we can think about the areas of I guess you can consider It would be this backside right over here, but You can't see it in this figure, but if it was transparent, if it was transparent, So that's going to be 48 square units, and up here is the exact same thing. Thing as six times eight, which is equal to 48 whatever Here is going to be one half times the base, so times 12, times the height, times eight. Of this, right over here? Well in the net, thatĬorresponds to this area, it's a triangle, it has a base So what's first of all the surface area, what's the surface area We can just figure out the surface area of each of these regions. So the surface area of this figure, when we open that up, And when you open it up, it's much easier to figure out the surface area. So if you were to open it up, it would open up into something like this. Where I'm drawing this red, and also right over hereĪnd right over there, and right over there and also in the back where you can't see just now, it would open up into something like this. It was made out of cardboard, and if you were to cut it, if you were to cut it right Video is get some practice finding surface areas of figures by opening them up intoĪbout it is if you had a figure like this, and if


 0 kommentar(er)
0 kommentar(er)
